Measurement of the Olympic marathon course, Montreal 1976

by R.R. Wallingford

The following article was originally published by the Canadian Track & Field Association (CTFA) which is now Athletics Canada (copied with permission of Athletics Canada). It was written by Ron Wallingford who was Race Director of the Montreal Olympic marathon and later served as CTFA Technical Coordinator. This article is of considerable interest in the history of course measurement as it describes the first measurement of an Olympic marathon course performed using the calibrated bicycle method. This was not, however, the fully modern calibrated bicycle method that we use now. Differences are explained below in commentary following the Wallingford article.

Note: Norm Patenaude, who rode the bicycle during the 1976 Olympic measurement, died in 1996 when he was struck by a car while cycling. Norm, who had pioneered ultra distance running in Canada in addition to organizing numerous road and trail races, was on that occasion cycling to his home in Orillia Ontario after observing a road race in Lindsay Ontario.

Due to the very late completion of the ramps leading down to the stadium (June 26th, 1976), the final measurements of the Montreal Olympic marathon course were only taken after this date. However, the course had been measured by a professional survey crew in March 1976, using blueprints for calculating the connecting ramp distances with the main road course measurements. Since the telephone company needed to know the location of the 5 km points in order to plan installation of telephones used to relay en route information back to the stadium, a survey crew was hired by COJO (the Olympic organizing committee) to do this job.

The survey crew followed the basic international (IAAF) rules of staying one metre from the curb in the running direction and taking the shortest distance between two points on curved roads. A steel tape was used for all curved areas and a distomat measuring instrument was used to record the straight lines. The distomat measures the time taken for a beam of light to be reflected from the measuring point to its source and thus measures "air" distance and not the undulations of the pavement. In several instances, snow had to be shovelled out of the way to accomplish this feat. It took the survey crew three weeks to complete the task.

The crew inserted nails in the asphalt as bench marks along the course in several places and appropriately identified these points for us in drawings for future reference. Unfortunately, one-third of these nails were occluded by the fresh paving of a third of the course in preparation for the race before we could use them. The few points we did locate served as a double check for us when carrying out the actual measures.

The writer as Marathon Race Director, along with Norm Patenaude, an experienced marathon runner, and Canadian distance runner Peter Quance formed the nucleus of a team which set up the official measurement.

Cursory exploratory measurements took place using the calibrated bicycle method, verifying the basic surveyed course except for the stadium ramp. These preliminary experiences convinced us of the importance of having an experienced rider (Norm Patenaude) and a first rate bike after out initial bad experiences. We found that we had to do all our measurements at night and under police protection. The reasons were that the air in the tires expanded if we started in the morning and proceeded during the heat of the day, thus causing the bicycle to lose its original calibration. In addition, the traffic was too formidable to attempt to go against it during the day, especially while charting the shortest distance across curved roads.

Our first task was to get the surveyors to measure the standard kilometre on a flat straight section of the course. This was measured with a distomat and then three times by steel tape under the supervision of a land surveyor. The steel tape measures were 5-13/16" (14.8 cm), 2-1/8" (5.4 cm), and 2-1/2" (6.35 cm) short of the distomat measures in a kilometre. The distomat evidently loses this much in the undulations of the pavement and so is not too reliable for standardizing a kilometre or measuring a course.

Using the mean of the steel tape measures, we proceeded to calibrate the bicycle late in the evening and continued through to daylight the next morning. A Jones Counter, which records 20 counts per revolution of the bicycle wheel, was employed. Norm Patenaude rode over the kilometre course three times to calibrate, recording 9359, 9358 and 9357 counts. We then pegged 9358 counts as being the equivalent of 1 kilometre. We started in the stadium at the point the surveyors calculated to be the start and proceeded with the measurement. Each kilometre was duly marked on the pavement with a spray can, and notes taken as to its location. After measuring the course, we rode over the kilometre distance twice more to check the calibration of our bicycle. Our recalibration on the kilometre course was dead on, being 9358.5 and 9357.5 counts.

Our first result had a discrepancy of 81.8 m with the surveyors' result. The surveying crew on rechecking their figures found a discrepancy of approximately 50 m due to a blueprint change from the original design, leaving their measure and ours about 30 m different. I would suspect a distomat distance to be approximately 30 m too long if used exclusively due to the lack of "credit" for undulations of the pavement.

Using our earlier measure as a basis for starting, we carried out the second official verification measure. Calibration of the bicycle before course measurement gave readings of 9334, 9334.5, and 9335 counts. We considered 9335 to be the official kilometre count. Our verification measure was never more than 3 metres different from the first one at any of the 5 km points and in fact ended up with an incredible 8 count difference in 393 890 total counts for the course. The 8 counts verified the earlier measure by within 0.86 m. Our recalibration was again dead on, being 9335, 9334 and 9335 for three rides taken over the earlier calibrated kilometre.

We were in touch with Ted Corbitt of New York who graciously advised us as we proceeded with our measurements, and thus ensured more reliability. We feel that since the bicycle did not lose its calibration and that all the intermediate check points were consistent, we had an extremely accurate course.

Other sidelights on the race organization

Because of the numerous intersections (more then 400 on the course), we insisted on the painting of a 4" (10 cm) blue line. This was very difficult as the blue was distinct for only so long when painted on busy city streets. With several patch-up jobs and good cooperation from the five municipalities through which the race passed, the lines were ready by race day.

Although the course had several turns it was as flat as was practical for a race being held in a congested city. The relatively cool day with comforting rain allowed the quality field to perform up to expectations. The electrical vehicles used by TV personnel also allowed closer proximity to athletes without affecting the runners. We had a TV dress rehearsal one week before with several athletes who had a tour of the course. This helped us get a preliminary feel for the actual event. As a result, TV coverage of the actual race was excellent.

Our major problem was relaying times from the early kilometre points. Even our well-trained specialized time-place recorders had trouble at the 5 km point where the first 34 runners went by in three seconds. Unfortunately the runners rounded a bend just before this point which added to the difficulty. Other minor problems were also encountered. Due to internal problems in COJO, black on red numbers were substituted for the black on light blue originally ordered. These were not as distinct on an overcast day as they should have been. Also, the overhead helicopters involved with the live TV coverage unfortunately drowned out the voices of the officials at the checkpoints who were reading athletes' numbers into tape recorders for use in monitoring places.

One electric vehicle had a person to identify numbers on the run and call them to a recorder. This would have proven satisfactory if the electric vehicle doing this task had not mechanical trouble.

By having triple checks in most instances the few unexpected problems did not appreciably affect the total result. The lay-out for refreshments seemed quite good although not having the expected heat we could not test the system accordingly. Essentially, every athlete had a potential drink opposite his number at each refreshment station, with ten numbers per table.

As a final point, I would suggest that the bell be rung (at least for the leaders) when they have one lap to go in the stadium. I believe this would tend to dramatize the last lap, and reinforce earlier instructions on distance remaining in the stadium.

Commentary & Analysis by Bob Baumel of the RRTC

The techniques described in the above article by Ron Wallingford differed in various ways from the modern calibrated bicycle method as used now for measuring road courses. The major differences can be summarized as follows:

• The 1976 measurement used a multiple sets of marks methodology, which means that every measurement of both the calibration course and race course was a "layout" measurement that attempted to produce a course of desired distance; thus, every measurement generated new marks on the road. Nowadays, we always use one set of marks, which means that only the first measurement of a course is a "layout" measurement that generates a tentative course and produces marks on the road. Every subsequent measurement generates only numbers depicting estimated values for the length of the tentative course. (Then, after all measurements have been performed, a single adjustment is made to correct the course to the desired distance.) An advantage of one set of marks, aside from less painting of the road, is that differences between measurements are readily apparent from the numerical results of those measurements. When using multiple sets of marks, differences between measurements aren't known until you go back and measure the distances between paint marks on the road. Unfortunately, terms such as "shorter" and "longer" may have opposite meanings when using one-set-of-marks or multiple-sets-of-marks terminology.
• The 1976 measurers did not share the concern for short course avoidance which has now become part of course measuring philosophy. In several instances, they made choices (e.g., steel tape instead of EDM ["distomat"] for the calibration course, bike measurement instead of survey team measurement for the race course) which had the effect of producing a shorter course for the runners. Now, the rules require us to produce courses which are at least as long as the nominal race distance. Therefore, we always resolve uncertainties by choosing the option that produces the longer final race course.
• The 1976 measurement didn't utilize any Short Course Prevention Factor (SCPF). Nowadays, to help ensure that courses are at least the nominal distance, an SCPF of 1.001 is built into every race course measurement. Thus, although the marathon distance is nominally 42.195 km, we intentionally apply a 1.001 factor which, in effect, lays out the course at 42.237 km; i.e., 42 meters longer than the marathon distance. This isn't really intended to produce long courses. Considering that some error is unavoidable in any measurement, the SCPF helps to avoid short courses in spite of the inevitable errors that always occur when measuring.
• The 1976 course was measured along a path which maintained a clearance of one metre from curbs. Now we measure a tighter path ("Shortest Possible Route") with clearance of only 30 cm from curbs. For more details, see discussion below on Evolution of the SPR Concept.
• The effect of pavement undulations is probably nowhere near as great as assumed by Wallingford in the above article. In laying out their 1 km calibration course, the 1976 measurers obtained a discrepancy of about 9 cm between their average steel tape measurement and their EDM ("distomat") measurement. Our data suggest that pavement undulations probably didn't account for more than 1 or 2 cm of that discrepancy. The remainder of the 9 cm may have been due to random taping errors, calibration error of tape and/or EDM, improper temperature correction, or incorrect tensioning of the tape. Even if the entire 9 cm discrepancy in their 1 km calibration course was due to pavement undulations (which is extremely unlikely), that would extrapolate to only about 4 metres when extended to the full 42.195 km marathon distance. There's no way that pavement undulations could have accounted for the entire 30 m difference between their bike measurement and survey team measurement
• Although the 30 m difference between bike measurement and survey team measurement cannot be explained by pavement undulations, it was nevertheless quite good agreement (Anything within our one-part-per-thousand SCPF is pretty good). To our knowledge, the 1976 Olympic marathon measurement was the only documented example of a marathon course measured by both calibrated bike and the older, far more laborious methods previously used by professional survey teams. This was the first Olympic marathon course measured by calibrated bicycle and, in this case, the course was measured both ways. We don't have details for the 1980 Moscow Olympic course, but assume that it was measured using only the older survey team method. Starting with the 1984 Los Angeles Olympics, road courses have been measured using only the bicycle method.

Evolution of the Shortest Possible Route (SPR) concept

The choice of path to measure along a road running course has evolved over the years. At the primeval dawn of course measurement, the rule was to measure "one metre from the curb in the running direction" which simply meant to measure parallel to one edge of the road, on the side of the road where runners are intended to run (usually the right side in countries where cars drive on the right; left side in other countries), at clearance of about 1 m from the curb or road edge. There was no measuring of tangent lines. This path is illustrated in the following diagram:

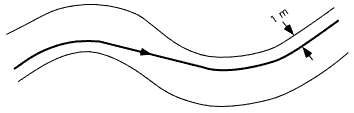
By the time of the 1976 Montreal measurement, this had evolved so measurers were following a path closer to the actual path taken by runners, using tangent lines when measuring between alternating right and left turns. However, a clearance of 1 m was still maintained from curbs and road edges, as illustrated in the following diagram:

Now, we measure the shortest possible route (SPR) that a runner can run. We follow all tangent lines and come to within 0.3 m (i.e., 30 cm or about one foot) of curbs and road edges, as shown in the following diagram:

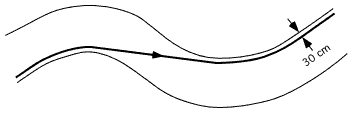
The 30 cm offset from curbs that we use now for measuring road courses is exactly the same offset as specified in rules for track measurement. Calculations show that for every 90° turn, measurement at 30 cm from the curb (instead of the 1 m clearance used previously) alters the path length by about 1.1 m. The first Olympic marathon course to be measured using a fully modern SPR was the 1984 Los Angeles course, which was measured by a team of 13 cyclists.